 ⇄
⇄
|
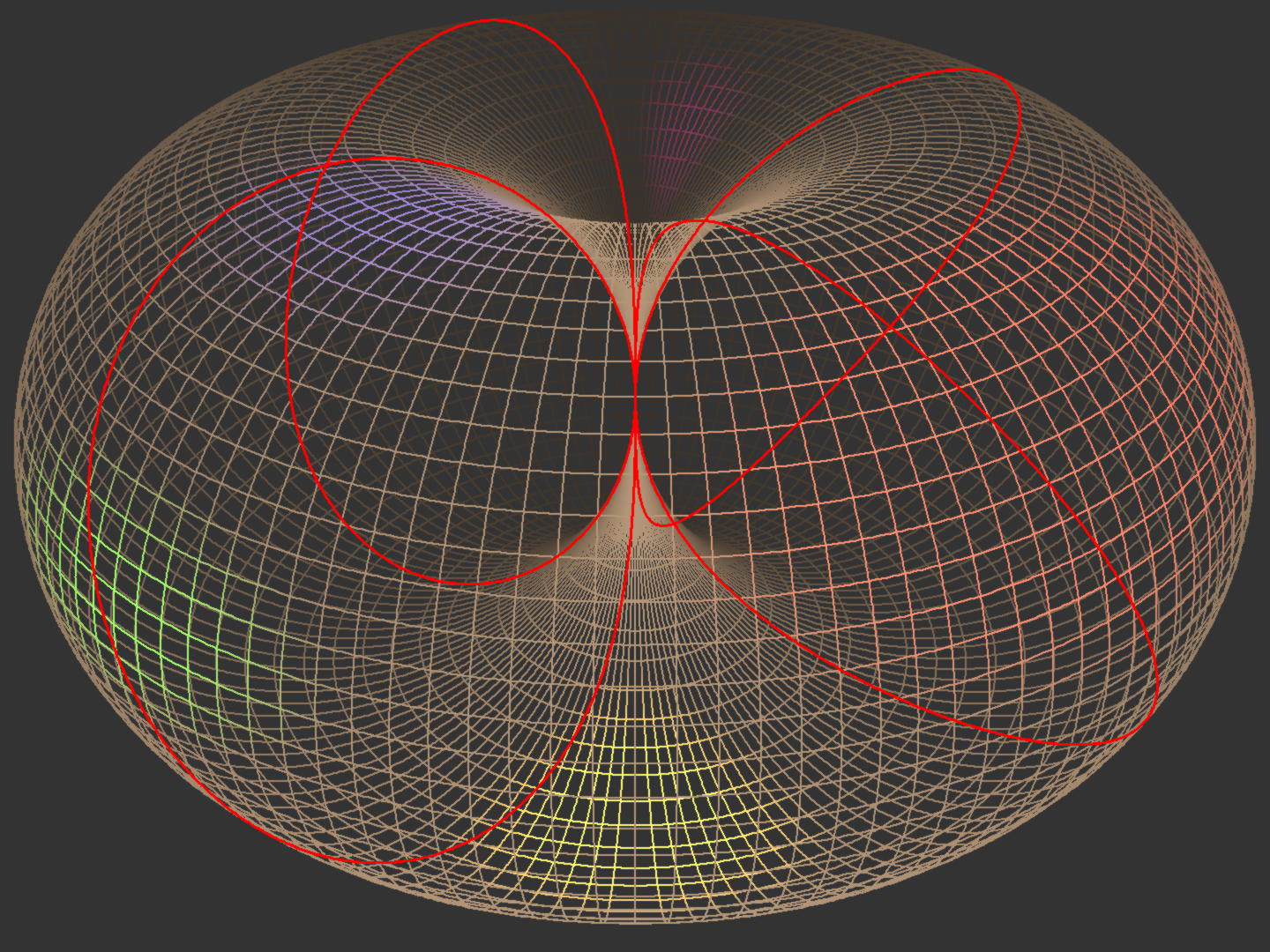
There is an important difference between the pictorial representation of of the set (body) of complex numbers by a static sphere
(Riemann) resp. a static horn torus (V. Puha) and my model. The first are a mappings* from the complex plane to surfaces within
a three-dimensional space, whereas 'my' horn tori are dynamic: they execute rotations around the main symmetry axis (real part)
and revolutions of the torus bulge around itself (imaginary part of the complex number). They change their size by revolution and they
are many, as many as 'particles' in our universe, all nested into one another, all intertwining at one ('spatial') point. They are
not embedded in any three- or more-dimensional space, but every horn torus 'entity' embodies one coordinate of an
infinite-dimensional space. The static horn torus is a rather simple figure with little properties. Its incredible complexity and
creative capability only arises when the dynamic is added, as described and illustrated on these webpages. ( visualisations e.g. explanation , trajectories , resonances ) Mathematically associable English texts e.g. can be found on pages starting here, but to treat the matter really mathematically, one first of all has to conceive the principle, what in my view only can be transported as colloquial speech. I know that is not the mathematician's way to cognition, but I treat it as a more philosophical and fundamental physical topic, though: 'my' horn tori represent complex numbers too - only differently. Their dynamic shows the capability of complex numbers 'quasi to calculate with themselves and mutually with each other' - as creators of our world. → front page / sitemap / previous page *) example for a mapping that preserves right angles between coordinates: |
 ⇄
⇄

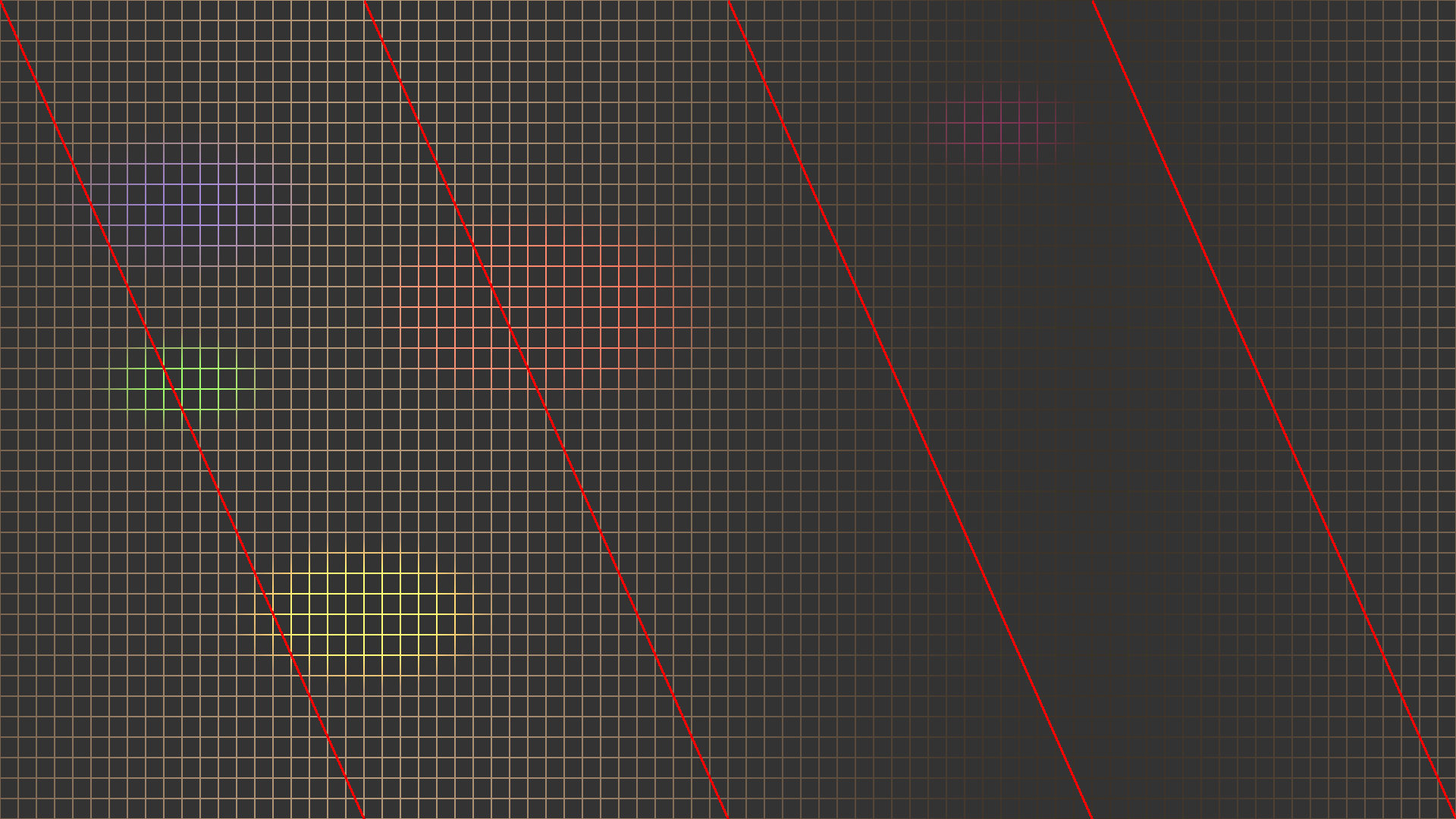
upper and lower edge of the plane both correspond to the center point of the horn torus (but note: it is not the complex plane!)
| horn torus latitudes maintain distance, while longitudes converge in vicinity of horn torus center - for full conformal mapping the latitude scale has to converge with same (sinus) rate as for
longitudes (on Riemann sphere: arctan),
otherwise we obtain deformations of structures and patterns as in this image , part of horn torus art , or - nicely seen - with an animated hexagonal pattern , but note: the structure-producing dynamic
horn torus is non-coformal ! (why should it be conformal?) |